Clocks and Mathematics

It is not very often that you see these two words together. But they are more intertwined than you can probably guess.

A major part of Mathematics deals with counting, and counting is exactly what clocks do. Clocks perform mod 12 and mod 60 calculations and therefore, modular arithmetic is often called clock arithmetic!

Clocks usually have an energy source which powers a shaft. The shaft rotates at a fixed rate, but this rate has to be adjusted to move the second, the minute and the hour hand individually. If you pry open an analog clock you would see something like this:

image taken from pixabay.com

The gears in a clock are used to control the speed of individual hands of the clock. Consider the image below. The smaller gear(green) has 20 teeth and the larger one has 60. Clockmakers use the term pinion for the smaller one and wheel for the larger.

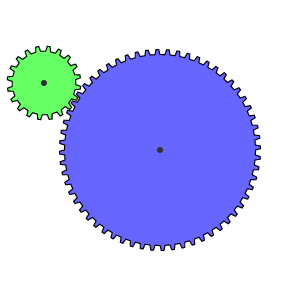
image source

When the small gear completes a rotation, 20 teeth have moved on the bigger gear, causing 20 teeth of the bigger gear to rotate. So 1 full rotation of the smaller gear gives 1/3rd rotation of the bigger gear. If our shaft rotates once a second, then to drive our minute hand we can use a pinion to wheel ratio of 1:60. Or, we can use a setup like the one in the next image. The green pinion turns the blue wheel which in turn rotates the red gear at the same rate. The green and blue gear combination reduces the speed by a factor of 1/6. The red wheel has 10 teeth while the grey has 100, thus further reducing the speed to 1/10th of the red i.e. the speed of grey wheel is 1/6 x 1/10 = 1/60th of the green. So we get a hand which moves once in 1 minute.

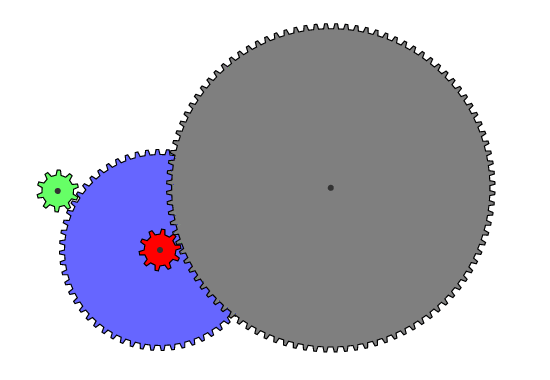
image source

This can be of course done in many other ways. Consider a hand which moves once every 12 hours. Then we would need to slow down the speed by 1/720th of the shaft which moves once in a second. While one can use a pinion with 10 teeth driving a wheel with 7200 teeth, larger number of teeth are not only more difficult to make but also larger gears need more energy. One can use a 3 stage gear train with the ratios of 10, 8 and 9, noting that:

1/720 = 1/10 x 1/8 x 1/9
The key here is that we can factor 720. What if we were to solve the problem that Camus had asked in the 18th century?
Suppose we have a pinion which rotates completely once in an hour. We need to use this to drive a wheel which needs to rotate once a tropical year i.e. 365 days, 5 hours, 49 minutes. Converting both the periods to minutes, we find that we need the ratio to be 720/525,949. The problem here is that 525,949 is a prime and we cannot factor it exactly to make a gear train of gears with smaller no. of teeth to obtain the same ratio. So we need to approximate it.

Brocot showed that we can find excellent approximations of any rational number using the stern-brocot tree. You can read more about it from the link in the reference. Using the Brocot tree, we find that 196 / 143,175 has a value very close to what we require. This can be factored as:

196 / 143,175 = 2/3 x 2/25 x 7/23 x 7/83
Thus we can use a 4 stage gear train to make the last wheel turn just 4/196 minutes less than a tropical year i.e. this is just about a second too fast, which is actually a very good approximation. Choosing 196 / 143,175 is favourable because it could be factored to relatively smaller numerators and denominators.

Not happy with this deviation by a second? This approximation can still be bettered using Brocot's tree given you can factorize it appropriately.

Reference
American Mathematical Society, feature column: Trees, Teeth, and Time