World record surfaces - 100+ follower special

Hey guys, not until recently did it occur to me that I have accrued over 100 followers.
This is amazing - and some of you are not even bots. 😋

Speaking of records: Did you know that records do not only exist in our mundane reality, but also in mathematics?

In algebraic geometry for instance you may challenge yourself to find a so-called record surface in the following sense:

Consider the complex 3 dimensional projective space P₃(ℂ), the space of all complex planes through the origin of the complex 4 dimensional vector space ℂ⁴.
Given an integer number d, find a polynomial p_d(x,y,z,w) of degree d such that the corresponding surface of all points X={(x,y,z,w)∈ℂ⁴|p_d(x,y,z,w)=0} has a maximal number of singularities.
By this, to put it simply, we understand points of X in which the regularity of the surface is in some sense violated, e.g. because the surface intersects itself.

While there are certain algebraical results yielding general bounds on the possible number of singularities depending on d, finding an actual surface attaining the maximal number (if it even exists) is an entirely different challenge.

One of the most interesting and in my opinion prettiest cases however is given by d=6 and the so-called Barth sextic. This surface, discovered by Wolf Barth in 1994 is one few representatives of record surfaces, by which the highest theoretically possible number of singularities, in this case 65, is realized.

A very nice Barth sextic in real 3D space, University Mainz

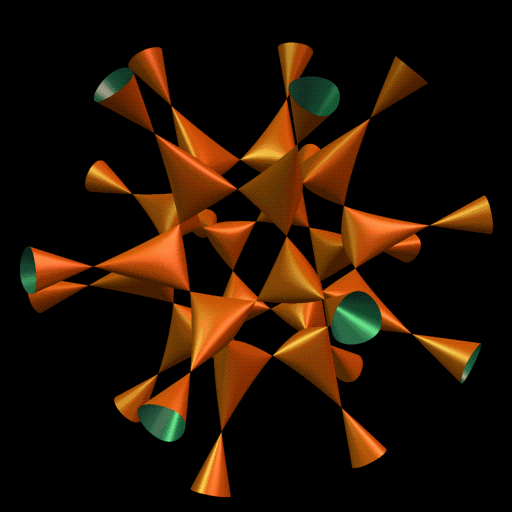
So not only are there no surfaces (given as a level set of a degree 6 polynomial) having more singulaities than the Barth sextic, but it also admits a particularly nice geometry as a projection into the usual 3D space (looking at the sub-surface obtained by setting w a positive constant) which you can see above.
In fact much of its aesthetic appeal derives from its symmetry group - It actually has the joint rotational symmetries of dodecahedron and icosahedron, the 12- and 20-sided dice with pentagonal and triangular faces which all of you know from your regular Dungeons&Dragons sessions I'm sure 😄

Sources:

• Barth's sextic, University of Mainz
• Weisstein, Eric W. Barth Sextic from MathWorld--A Wolfram Web Resource
• Barth, W. "Two Projective Surfaces with Many Nodes Admitting the Symmetries of the Icosahedron." J. Alg. Geom. 5, 173-186, 1996.

P.S.: If you count singularities in the picture you should obtain less than 65. This is not an error but a result of representing a mere "slice" of the entire surface in the much smaller 3-dimensional real space rather than considering the whole in P₃(ℂ).