How likely you will pass a multiple choice test without learning? - Binomial distribution basics

Binomial distribution tells us about how often a certain outcome which is either true (p) or wrong (p-1) will occur in "n" times of independently repeated experiments. A single success/failure experiment is also called a Bernoulli experiment and many Bernoulli experiments in a row are a Bernoulli process, named after the swiss mathematic Jacob Bernoulli.

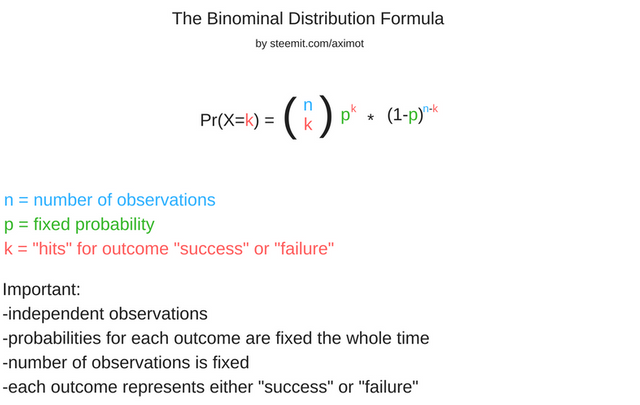
Edit: the heading should be: binomial and not "binominal"

Example:

A dice get's thrown 20 times. We know, for every number there is the same probability of p= 1/6. If we want to calculate how often the number "4" will occur if we throw the dice 20 times, we use the euqation from above.

Keeping in mind that a binomial distribution has only two possible outcomes and not one for each number, success is determined by throwing a 4 and failure by throwing all the other numbers (1,2,3,5,6). Our n=4 and is randomly chosen, it works with every other number aswell, depending on how often the experiment should be done.

The order of the success and failures is not relevant for our calculation:

(s= success, f = failure)

(s,s,s,f,f,f,f,f,f,f,f,f,f,f,f,f,f,f,f,f) -> ((1/6) ^3) * ((5/6)^3)

is the same as

(s,f,f,f,f,f,f,f,f,f,f,f,f,f,f,f,f,s,f,s) -> ((1/6) ^3) * ((5/6)^3)

-> This shows the independence of events. The probability of throwing a 4 is the same, no matter in which order the successes are.

p= 1/6 and (1-p)= 5/6

k= 4

n= 20

Putting all the information into the equation:

(n k) is stated as "n choose k" ( possible way of choose k hits from n observations)

Now an examplary situation where it could be very useful to know the binomial distribution ;) :

Surprise test! Waking up from you scheduled nap in history class, your teacher hands out a surprise test about the current topic. Having no clue what the topic even is, you remind yourself of something you heard of in math class called "binomial distribution". 

As soon as you get the test, you start to count the questions: 25. Possible answers for each question: 4. 

Having no clue who the 3th president of Ireland was, there is no other way then guessing the solutions left.

So how can we know how possible it is for the person to pass the test?

We know the parameter n= 25 -> numbers of questions and p= 0,25 -> 25% chance to tick the right answer. Let's say the pupil has to have 10 questions of the test ticked right to pass it (k= 10). Our equation tells us about the probability he ticks exactly 10 times a right answer. Another approach would be to say the pupil has to tick at least 10 answers, meaning we would have to subtract the values of k < 0 from 10 = + k > 10. To keep it simple, we just use k = 10:

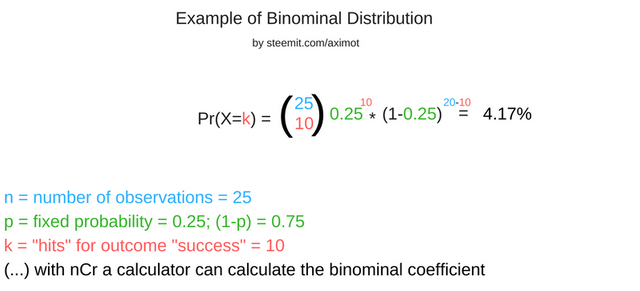
As we can see, ticking 10 out of 25 times the right answer wouldn't be a good strategy. Better search for a possible (:D)  excuse to escape the test and spend some time learning on it, it increases the chances drastically.

Have a nice day :)

Sources

Text

https://en.wikipedia.org/wiki/Binomial_distribution

http://www.statisticshowto.com/binomial-distribution-formula/

http://www.stat.yale.edu/Courses/1997-98/101/binom.htm

https://de.serlo.org/mathe/stochastik/wichtige-modelle-verteilungen/bernoulli-kette-binominalverteilung/aufgaben-bernoulli-kette-binomialverteilung   (translated)

Pictures

All pictures were made with Canva.com