Engineers of yesterday allow us to move forward in the now, a brief historical look
Engineers of yesterday allow us to move forward in the now
a brief historical look
The Civil Engineering and especially its structural branch from which emerges the resistant earthquake engineering , always remains in the quest to conceive, design, project, build and maintain works and constructions that allow its users to develop their daily activities. In this sense, as a lover of history, I value all the effort and work developed by previous professionals that have allowed me to reach the current level of the state of the art of my profession, which currently allows us to reach ever greater heights.

In this sense, there are few current textbooks of structural analysis that include some historical perspective. In fact, many of them visualize structural engineering as a simple application of science and expressions mathematics conceived and formulated by scientists and mathematicians for centuries.
While it is true that a large part of the theoretical expressions used and applicable in an area known as elastic zone are more than 100 years old, many important theories were conceived and formulated by engineers. They developed these concepts and methods, not as theoretical abstractions, but to solve real engineering problems. As engineers, their goal was not simply to explore, but to create, solve and innovate. Therefore, below are some biographies of the engineers that, in my opinion, had a profound impact on the progress of structural engineering.
Charles Augustin Coulomb (Angoulême, Francia, 14 de junio de 1736 - París, Francia, 23 de agosto de 1806)
Charkes Agustin Coulomb was born in France in 1736. He received his engineering education at the Ecole du Corps Royale du Genie. Shortly after graduating in 1763, he was assigned to the Island of Martinique in the Caribe Sea, where he was responsible for the design and construction of some fortifications on the island, among other projects.
.jpg)
Being a young officer of military engineering, Coulomb soon realized that he did not understand the true behavior of the structures. This led him to analyze a series of structural problems in Martinique, including the curvature of beams.
In 1772, Coulomb returned to France and during the following 10 years undertook several engineering projects. In 1773, he presented his now famous Elastic Theory of Bending to the French Academy. Work by which for many engineers, Coullomb can be considered as the "father of structural analysis", because he was the first person to formally affirm that the beams must satisfy three equilibrium equations, he reasoned that all internal forces acting in any section of the beam must be zero.
For example, for the sum of the horizontal forces to be equal to zero, he reasoned that the internal tensile force in a given cross section should be equal to the internal compressive force. Similarly, for the sum of the vertical forces to be equal to zero, he thought that the internal shear force in a given cross section should be equal to the applied vertical loads. And finally, for the sum of the moments to be equal to zero, he reasoned that the internal moment in a given section should be equal to the moment caused by the charges in the section. Coulomb also correctly assumed that the initially plane sections before bending curvature remain plane during flexion and assumed that typical beams could be considered in general as perfectly linear and elastic.
His reasoning led to the expression of the theory of elastic flexion, established in modern terms as σ = M / S, where σ is the effort to bend, M is the moment of bending that acts on a particular section of the beam, and S is the elastic section modulus is defined as S = I / ymax, with I like inertial module a and ymax as the distance from neutral axe to the edges of the cross section.
Louis Marie Henri Navier (Dijon, 10 de febrero de 1785- París, 21 de agosto de 1836)
Louis Marie Henri Navier was born in France in 1785 and was raised by his uncle, Émiland Gauthey, a famous engineer. He graduated from the Ecole Polytechnique in 1804 and the Ecole des Pontes et Chaussees in 1808.
After the untimely death of his uncle 1807, Navier began his career undertaking the monumental task of completing his three-volume work on bridges and canals. Although Navier occupied most of his time in theoretical work, he also practiced engineering, generally designing or building bridges.

In 1819 he was able to determine the zero line of mechanical stress, now known as neutral axis or neutral fiber. Finally correcting the incorrect results of Galileo Galilei in 1826 he established the elastic modulus as a property of materials independent of the second moment of the area better known as Inertia.
In 1824, Navier joined the faculty of the Ecole Polytechnique and, in 1836, published the first textbook dealing with structural analysis. In his book, Navier used the laws already formulated by Coulomb on elasticity in beams and previous calculations made by Euler, to establish that the curvature experienced by a section of an elastic beam is equal to M / EI, where M is the moment of bending, E is the modulus of elasticity or Young's Modulus, and I the moment of inertia of the cross section. More importantly, he was the first to state that, for small deflections, the curvature is approximately equal to the second derivative of the deflection, d2y / dx2 = M / EI.
Navier, used this equation to determine the deflections of various configurations of statically determined beams. he also used the equation to determine redundant reactions of various configurations of statically indeterminate beams. Therefore, he was the first engineer to formulate and apply what we now call the Double Integration Method, and in a broader sense, he was the first to employ a version of the structural analysis strength method posed by James Clerk Maxwell (1831-1879) and refined by Christian Otto Mohr (1835-1918).
Benoît Paul Émile Clapeyron (Paris, 26 de Febrero de 1799 – Paris 28 de Enero de 1864)
Born in Paris, B.P.E Clapeyron was a French engineer and physicist, recognized by many scientists as the father of thermodynamic theory. He graduated from the Ecole Polytechnique in 1818 and from the School of Mines in Paris in 1820. Clapeyron helped to found the Institute of Engineers of the Forms of Communications, in St. Petersburg, Russia. In addition to his role as a professor of mathematics, physics and engineering, he also helped the Russians with the design of several important structures, particularly suspension and multi-span bridges.

In 1831, Clapeyron returned to France and worked in the design and construction of the French railways, being one of them the railway that communicated Paris with Versailles and Saint-Germain-en-Laye. In 1844, he joined the Faculty of Ecole des Pontes et Chaussees, where he shared his practical experience and his great theoretical knowledge.
In 1848, he reformulated the equations presented by Navier in terms of equations and unknown moments to then write a set of equations, which could be described as slope deviation equations (rotation differentials, from which elementary moment-rotation expressions can be generated) resulting in the theorem known as the three moments (or Clayperon's theorem) very useful in the calculation of hyperstatic continuous beams. That is, in general, Clayperon was the first person to use the Displacement Method of Structural Analysis.
Christian Otto Mohr (Wesselburen, 8 de octubre de 1835 - Dresde, 2 de octubre de 1918)
Mohr, was a German civil engineer, one of the most famous of the nineteenth century, and today most civil engineering students have used the work of Mohr regarding calculation of moments of inertia or effort on inclined axes, better known as Mohr circles.
Mohr studied at the Hannover Polytechnic. And at the beginning of 1855, during the beginning of his professional life he worked on the design of railways designing some of the first bridges and working with some of the first steel truss, applying the calculation method perfected in 1863 by August Ritter and known as the method of sections.

1867 Moh for his scientific achievements was so popular that he was proposed to the Polytechnic of Stuttgart (predecessor of the University of Stuttgart), where he became Professor of Applied Mechanics and in 1873 at the Polytechnic of Dresden. He was able to present the theoretical material of mechanics in such an easily understandable form, that his lectures were very well attended and later even published in autographed form.
It is also meritorious, the development of a graphical method developed to determine the elastic line as a catenary curve, which until then was only mathematically possible by double integration, this view of the problems makes use of Green's theorems (although they are commonly called theorems of mohr) and led to what is now known as the method of elastic weights and conjugate beam. In 1874, Mohr formalized, until then only incipient, the idea of a statically indeterminate structure refined the procedure established by Maxwell.
Hardy Cross (Nansemond Virginia 1885 – Virginia Beach 1959)
He was an American structural engineer, famous for being the creator of approximate and iterative methods of calculating statically indeterminate structures and analysis of hydraulic networks commonly known as Cross Methods.
He earned his Bachelor of Science degree in civil engineering from the Massachusetts Institute of Technology in 1908, and then entered the bridge department of the Missouri Pacific Railroad in St. Louis, where he remained until 1909. In 1910 he started his studies at Harvard where he obtained the title of MCE in 1911.

The method of Cross or distribution of moments, was used frequently between years 30 and 60, until with the advance of the computers was replaced by other methods based on the development of equations in the form of matrices known as matrix methods. The Santiago Bernabeu stadium where Real Madrid plays was analyzed using the Cross method. Although at the time there were other iterative methods such as those developed by Gasper Kani (1910 - 1968) and F. Takabeya, the Cross method was always the most popular both academically and professionally.
The Hardy Cross method is essentially Jacobi's method applied to the displacement formulas of structural analysis and applying the theorems of Albert Castigliano (1847-1884). Hardy Cross developed it while working at Harvard University. He then worked as an assistant professor of civil engineering at Brown University, where he taught for 7 years. Later in 1921 after a brief period in engineering practice in general, he accepted a position as professor of structural engineering at the University of Illinois at Urbana-Champaing. It was at this University where the method saw its final versions.
Another method of Hardy Cross is famous for modeling flows of drinking water supply network. Until recent decades, it was the most common method to solve such problems.
Conclusion
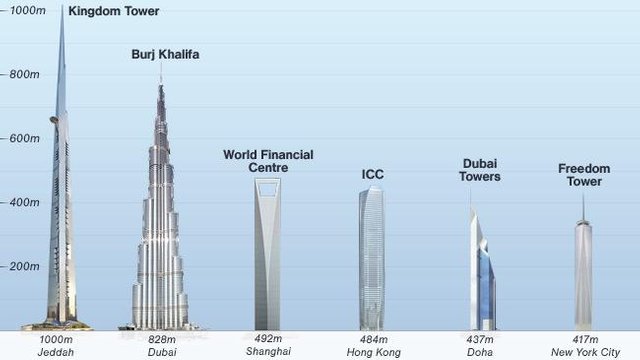
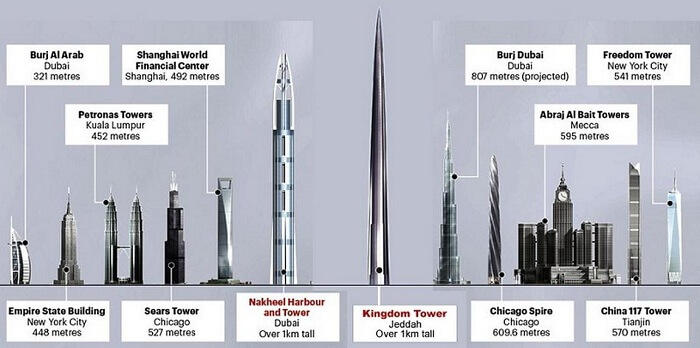
These brief biographies are exposed with the purpose of making known to the students of civil engineering and even to other types of readers, that the advance of the engineering has not only been given by mathematicians or scientists, but also by engineers who looked for the form more rapid, rational and safe practice of realizing the structures they designed or built, for which they first conceived and formulated the fundamental theories and methods of structural analysis, which led to the search that every civil engineer seeks to realize the good of humanity and that without their contributions buildings like the Jedahh Tower (under construction), the Burj Khalifa, the Taipei 101, the Petronas towers today would not be possible.
Bibliography
The history of the theory of structures. From arch analysis to computational mechanics by Karl-Eugen Kurrer
Structural Analysis: A historical perpeace by Frederick M. Law
The Science of Structural Engineering. by Heyman, Jacques (1999).
Until another article, I hope you liked it, if that was the vote and follow me @ davidg83