Knowing the math - Order of Operations Basics

Welcome to lesson on order of operations.

Let's take a look at it what we are going to do today is we're going to figure out what is order of operations and we'll talk specifically about two parts of the order of operations the exponents and the parentheses. Those parts attend to give us a little bit more trouble in future math classes so let's go ahead and get started..

The order of operations is basically a pattern that tells us which order we do math problems so we start off with parentheses anything that are inside of those brackets, parentheses grouping symbols whatever you'd like to call them whatever is inside of their gets done first, then we move along to our exponents, then we would move along to our multiplication and division I put these on top of each other now because they get done in order in one step in the order from left to right as they appear and I'll show you that in a couple examples today same thing with addition and subtraction they get done in the order they appear from left to right, so this is the basic order of Operations.This is the order of operations right here.

Let's actually do some questions using the order of operations and you'll see how they work. So the order of operations is basically that you would follow these four steps in order so we start out at looking at this problem 3 plus 2 times 5 which do we do first 3 plus 2 or 2 times 5

Well the order of operations tells us the correct order to do it look at it. First, we would look for parentheses I don't see any parentheses are no brackets or grouping symbols at all then I look for exponents we'll talk a little bit more about what those are later this question has addition and multiplication so let's go on to our next step multiplication comes first notice that we went parentheses, then exponents, then we hit our multiplication, division step so our multiplication and division would get done in this first step in other words, that's what gets done first so before we do 3 plus 2 we would do 2 times 5 which gives us 10.

Now the operation that's left over is 3 plus 10 or edition and that would get done in our final Step

So that's how the order of operations works you have to do it in this order first, we do are multiplying then we do are adding.

Let's look at another example, this one here has three operations, it as subtraction, division and multiplication, so let's go ahead and start solving it.

What are we going to do first, well our order of operations, says we don't have any parentheses or exponents so let's move on we would do our multiplication and division first so we start at the left and move to the right we do the first multiplication or division that we come to in this case it's 10/2 which gives us 5

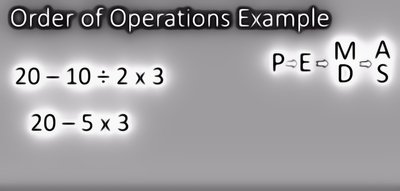
Then we still have a multiplication to do so, we would do 5 times 3 which gives us 15

Notice it wasn't first multiplication than division it was multiplication or division, whichever comes first working from our left to our right and then after all of the multiplication and division are done, then we move on to our addition or subtraction which in this case is subtracting 20 minus 15 which gives us 5

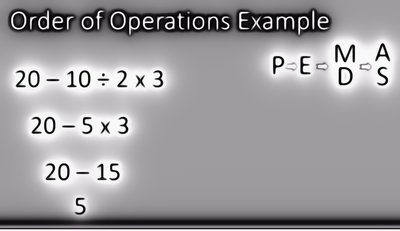
Doing it in any other order would give us a funky answer that's not what we're looking for, this is the correct way to do the order of operations.

Let's move on to another sample question here 3 squared plus 1 times 7 hold on there.

What is that this 3 with the two raised up there I called it 3 squared or 3 to the power of two what's that.

Let's talk about it.

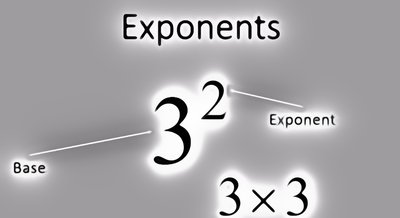
This is an exponent an exponent is when you have a base raised to the power of an exponent 3 to the power of two what it means is 3 times 3, how did where did that other three come from you multiply the base times itself and the exponent tells us how many times were writing down that number so it's written down twice. Let me show you an example that will hopefully help to clarify that.

2 to the power of 4 that is in exponent form.

So if I'm going to expand that out i would write it as the base 2 times itself and it's multiplied times itself four times so it's 2 times 2 times 2 times 2 notice in expanded form we can see clearly all. That's what 2 to the power 4 means

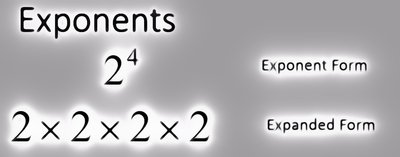
And then in standard form, we just simply write it out two times two is four four times two is eight eight times two is sixteen so that would be our final number in standard form

That's how exponents work you have the exponent form the expanded form and the standard form.

So now let's go back to our question 3 to the power of 2 plus 1 times 7

Let's use our order of operations to solve this question first there's no parentheses so we're going to come to our exponents so first we're going to solve 3 to the power of 2, 3 to the power of 2 is 3 times 3 which gives us nine remember it's the base times itself twice

So now everything else remains exactly the same look at plus 1 times seven so we finished the exponent part

Now we have to move on to multiplication and division first we're going to do our multiplication because that's what we have, we don't have any division so we'll do 1 times 7 which gives us seven

And now we'll work on our addition, subtraction 9 plus 7 gives us 16 so our final answer for this question of 3 to the power of 2 plus 1 times 7 is our answer will be 16

Alright, let's do another order of operations question 4 to the power of 2 plus 2 times the quantity of 7 minus 3 what is that .

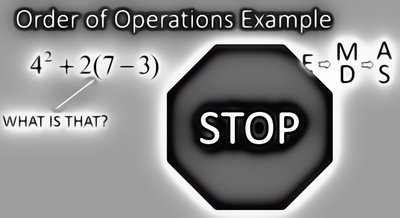
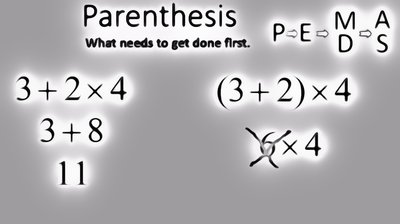
And that's what we're going to talk about. Next this is our parentheses parentheses are just grouping symbols those brackets or parentheses that show us what needs to get done first let me show you an example of parentheses in action 3 plus 2 times 4 that is a pretty straightforward order of operation question.

We would do the multiplying first 2 times 4 is eight and then the addition 3 plus 8 gives us 11

Now if we add parentheses in there through the quantity of 3 plus 2 gets done first and watch how it changes our answer 3 plus 2 gets done first that gives us six and we're multiplying it times 4. All right now are you paying attention because 3 plus 2 is definitely not 6 is 5 alright

I did that to see if you were paying attention.Alright 3 plus 2 is five and five times 4 gives us 20

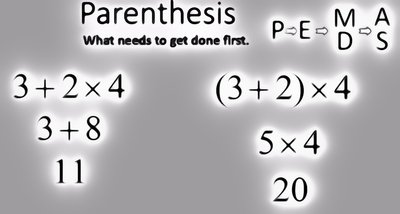
Notice how adding the parentheses change the order that we did things and it changed our end result so we have to keep our eye out for parentheses and do what's inside of the parentheses first and then do the rest of the operations.

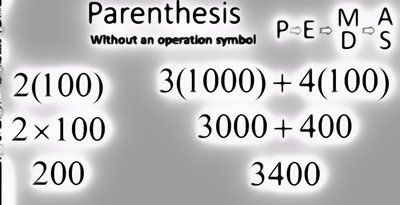
Now there are some situations where you get a parenthesis and there's no symbol like this 2 and 100

Whenever there's no symbol given it means multiplication that's the same as saying 2 times 100 which will give us 200

Here's another example 3 times 1000 plus 4 times 100 I'll just do the multiplication there, that means 3000 plus 400 or in other words 3400

Let me give you a little another, even longer answer your question 5 times 1000 plus eight times 100 plus 9 times 1

The way I'm saying it should tell you that it is multiplication, but whenever you're given parentheses without a symbol, it means multiplication this is 5,000 plus 800 plus 9 which gives us 5809

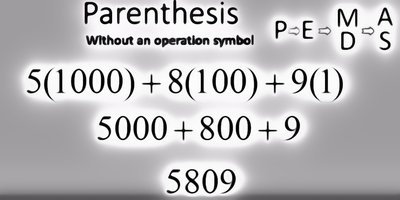
kind of a ridiculous question I know, but just an example to kind of show you how that works when you don't have the symbol.

So back to our question that confused us a minute ago.

Here we go let's go ahead and solve this, this is an order of operations question that has everything in it. We're going to start with parentheses what's inside of our parentheses or grouping symbols 7 minus 3 gives us 4

Notice I've written in the multiplication symbol so it's 2 times 7 minus 3 in other words 2 times 4.

Now I'm going to do exponents which is 4 to the power of 2, 4 times 4 which
gives me 16

Now I'll work on multiplication 2 times 4 is 8 and my addition is the last step 16 plus 8 it gives me 24.

So I just want to recap everything, this is a complicated order of operations question right here that has exponents parentheses multiplication and addition it, basically this type of question will help you to practice every part of those order of operations.

To a quick recap of everything that we learn.
We talked about order of operations are parentheses exponents multiplication, division than addition, subtraction we talked about exponents remembering that our base is multiplied times itself and the exponent tells us how many times are multiplying it and then our parentheses are silly question that we put together with parentheses.

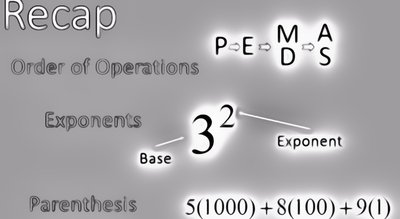
That's what we've talked about today I hope that lesson was helpful for you.