The Most Beautiful Equation in Nature
Let's discuss one of the most famous equations in mathematics. The equation reads:

This equation has been compared to a Shakespearean Sonnet, and has been called "the most remarkable formula in mathematics" by Nobel laureate Richard Feynman. Unfortunately, mathematics is usually despised (commonly due to some traumatizing tests in high-school), and so equations are not typically thought of as beautiful.
Let's try to understand why this equation is considered so marvelous.
The Components of the Equation
The equation is comprised of 5 numbers:
The number 0
The number 1
The imaginary number i
Euler's constant e
The constant Pi
Let's go through them one by one.
The Numbers 0 and 1

These are the simplest ones, but they are extremely significant, since these two numbers hold a special meaning for mathematicians.
On a daily basis, we have a very simple understanding of what numbers are. Mathematicians can't stand simple things, and so they had to make this concept much more complicated! The set of all of the "usual" numbers is a special case of what mathematicians call a "field". Simply put, a field is a more abstract form of the set of all numbers. Another example of a field is set of all possible fractions).
Now, it turns out that two numbers always stand out in any field. Both 0 and 1 have to appear in every single field. This sets 0 and 1 apart from the rest of the numbers, and gives the two numbers a special meaning for mathematicians.
These two numbers are also set apart in other fields in science, the most prominent example being the usage of binary numbers in computer science.
The Imaginary Number i
This number is a bit more interesting. The origin of this number is again the mathematicians' need for complexity. Mathematicians asked themselves, "how do we take the field of all of the numbers, and make it more complicated?" The answer was to invent a new number to add to this field, which was defined by the equation i^2=-1.
This might sound extremely odd - how can you invent numbers? But the truth is that this has been done over and over again throughout history!
We are used to calling everything "numbers": positive numbers, negative numbers, fractions, irrational numbers, and so on. But if you consider Roman numerals, you will notice that not all of these numbers exist in roman numerals. In fact, only positive integers can be described with Roman numerals. This means that other types of numbers weren't used as much.
This makes sense - a long time ago, these were the only numbers we had use for; no one ever wanted to buy "negative one apples" from someone else, and so the negative numbers were only added later on.
Studying the history of numbers, we see that we started off with only the positive integers, and then went on to add the number zero, fractions and negative numbers later (the order in which they were added varied in different cultures). Finally, irrational numbers were added to create the set of all of the numbers we agree upon today.
So it seems as though inventing numbers has been done more than once throughout history. The only reason why we think that the imaginary number is odd is because it was added much later on. In the same sense, thousands of years ago people probably thought that negative numbers were "odd", and it probably took them some time to get used to them.
Regardless, the number i is now very commonplace in mathematics. It is even an integral part of quantum mechanics - so integral, in fact, that some scientists claim that quantum mechanics cannot exist without it, which means that nature decided to give special meaning to the number i for some reason...
The Constants of Nature: e and Pi
The last two numbers are referred to as "constants of nature". It seems as though nature has singled out these two numbers, and made them special somehow.
Let's explain why this is so. First, let's talk about the origin of Pi.
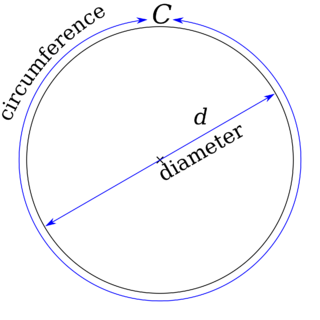
Let's do an experiment: draw a circle, and calculate its circumference. Now calculate its diameter. Finally, if you calculate the ratio of the two, you'll see that it is equal to Pi (aproximately 3.14).
This sounds completely unremarkable, until you realize that this is true for all circles. You can draw any circle, and the ratio of its circumference to its diameter will again be Pi.
Why has nature decided that this ratio be specifically Pi? Why isn't it some other number? What makes Pi so special? These questions have so far gone unanswered.
Next, let's talk about the number e (which is approximately 2.718). This number was originally encountered in the study of compound interest, which you can read about here. The constant e is also very significant in calculus, since the Exponential Function e^x is the only function whose derivative is itself. This seemingly random fact is actually extremely important, and is the reason e appears everywhere in nature (for example, when cells multiply, the function which describes the total number of cells is the exponential function).
Back to the Equation
The equation doesn't look as scary now, does it?
Now that we understand each component, we can understand why this equation is so significant. There are two main reasons one can point to:
This equation relates the five important numbers we discussed. This is extremely surprising! Each one of the numbers is significant in its own right, and is independent. There is no reason to think that we should find and sort of relation between these seemingly unrelated numbers.
This equation relates only the five numbers we discussed. One of the tests done in physics and mathematics to judge a theory is to check how simple and elegant it is. A theory which is elegant will have a much better chance of being agreed upon. This equation passes the test easily - it is extremely, extremely simple, which makes it attractive to mathematicians.
Bottom Line
Simplicity has always been an important test for any mathematical theory, and this equation is so simple that it immediately caught many mathematicians' eyes. The elegant way in which it relates these five important numbers is indeed remarkable, and it is certainly debatable whether it is comparable to one of Shakespeare's sonnets (Shall I compare thee to Euler's Identity?)
Not only the equation is simple and elegant, but also your words that describe this phenomena in such an understandable way. :)
Please, do also consider adding the sources for your images. A link were you found them is what would be cool.
The markdown for sourcing could be like this: [Image Source](put here the link)
the it looks like this: Image Source
You can also check this valuable tips from Steemprentice
And it would be highly appreciated to use photos "free for reuse".
Have a nice day! :)
Thank you for your notes, I've edited the post accordingly making sure to use "free for reuse" photos.
Thank you very much! It's appreciated a lot.
Can't not upvote Euler's identity!
I tried some different approach recently to arrive at this equation ;)
https://steemit.com/mathematics/@mcsvi/intuitive-math-everybody-can-understand-complex-numbers-part-1-it-s-all-about-rotation
Your post is awesome, and very understandable!
great job!
You posted it already here. Why a repost?
Beautiful explanation. Most people aren't aware of the beauty and importance of Euler's formula. You might enjoy this 10 minute video:
https://steemit.com/mathematics/@point/imaginary-numbers-breakthrough-to-reality