Combining Like Terms in Algebraic Expressions

I wrote an article on combining like terms in algebra long ago and want to share. We need to know the basic algebra, such as variables, coefficients, algebraic expressions and polynomials to be able do it. In this representation I am going to explain how to combine like terms in a polynomial or an algebraic expression.

Image Source

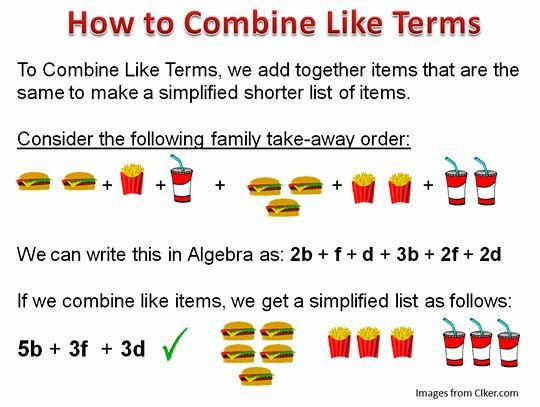
Like terms in a polynomial contain the same variable. For example “3a” and “6a” are the like terms because both terms contain the same variable “a”. On the other hand “3a” and “6b” are not the like terms as they contain the different variables “a” and “b”.

To combine like terms first of all the terms with the same variable are written together and then the coefficients are added or subtracted according to the integer rules. For example; look at the algebraic expression below:

2m + 7m

The above algebraic expression is a polynomial and it has two terms. But, both terms has the same variable, hence thy are alike and we need to collect both the terms (I mean we need to combine both the terms) to make one term. 

To combine 2m and 7m; we need to take a look at the coefficients and their signs for both the terms. Both th terms have positive coefficients; hence add both the coefficients 2 and 7 to get the new coefficient 9. 

Note that the variable stays the same as explained below:

2m + 7m

= (2 + 7) m

= 9m

Hence we have combined 2m and 7m (two like terms) to get 9m as the answer. 

Now question arises that when should we classify the polynomial; before combining the like terms or after combining the like terms?

Always, always combine the like terms before you classify the polynomial. In our example; the polynomial 2m + 7m, we can’t say it a binomial but it is a monomial because it has only one term and which is equal to 9m.

More examples to explain combining the like terms:

1) 3a + 8a + 4a – 11a

    = (3 + 8 + 4 – 11) a

    = (15 – 11) a

    = 4a

2)  8m + 5n – 6m – 9m 

      = (8 - 6 -9) m + 5n

Notice that “8m, - 6m and – 9m” are all like terms, but 5n is different term, hence kept separate.

= (8 – 17) m + 5n

= - 9m + 5n

3) ab + 5a – 7a + 9a + 4c + 11ab – c 

This polynomial has three kinds of different terms; terms with variables “ab, a and c”, collect the like terms as shown below:

= (1 +11) ab + (5 – 7 + 9) a + (4 – 1) c

= 12ab + 7a + 3c 

Keep in mind that the terms “ab” and “- c” have coefficients “1” and “-1” respectively.