Hi steemers, @federicopistono here.

Today I'm publishing on Steemit in full the third chapter of my book Robots Will Steal Your Job, But That's OK, an international success published in seven languages, featured on Forbes, the BBC, The Wall Street Journal, The Economist, New Scientist, CNBC, and was subject of discussion at numerous universities as well as the European Commission, the Nobel Peace Center and the Italian Parliament.

Enjoy!

Chapter 3: Exponential Growth

One of the most important, yet misunderstood concepts in our lives, is the nature of the exponential function. You may have heard of this term before. Maybe it was mentioned in some newspaper article in the technology section, briefly cited and hardly explained at all. Or perhaps under the name “compound interest" when you took out a loan from your bank. Of course, they usually tend to gloss over its real significance, and rarely does someone explain what it really means. Yet, it pervades every facet of our lives, the economy, and the decisions we will have to make in the future. Understanding the power of the exponential function is key in proceeding further with the analysis presented in this book.

Albert Bartlett, Professor emeritus of Physics at University of Colorado-Boulder, during a very famous lecture he gave, stated that “the greatest shortcoming of the human race is our inability to understand the exponential function.”[1] This is no light statement. Professor Bartlett has lectured over 1,600 times since 1969 on Arithmetic, Population, and Energy, trying to warn as many people as possible about the dangers in failing to understand this most important concept.

Before the end of this chapter, I want you to have a clear understanding of the exponential function. It does not matter whether you have a degree in philosophy, in economics, if you are a college dropout, if you are uneducated, unemployed, if you are a Professor at university, or the CEO of a multinational corporation; chances are you do not fully understand what exponential growth really means. Yet, it is imperative that you do.

I’ve given many lectures during my life, to all kinds of audiences. Even among the most educated ones, people fell short when confronted with very simple examples of exponential growth. -However, when properly explained, everyone was able to understand it. This gives me hope, because it is crucial that everybody realises what it means, and what the consequences are of applying steady exponential growth in the years to come.

Enough with my ramblings, are you ready? Good. Let us dig in and see what it is all about.

The exponential function is used to describe the size of anything growing steadily over time. For example, suppose you have to buy a house, and the bank gives you a loan at 7% interest. What it means is that every year the amount of money you have to give back grows by 7%. The first year the quantity grows by a tiny amount (turning the debt to a total of 107% of the principle), but on the second year it grows relative to the last amount, not to the original principle. So, 7% of 107%. The following year it grows even more, and so it goes. Can you guess what will the amount be in 20 years? Not too easy, unless you have taken statistics in college. It is not my intention to explore the mathematics of the exponential function (although it is really interesting and I suggest that some of you do). I want you to understand it in very clear and effective terms, so I will give you a simple formula that you can use any time, anywhere, and all you need is first-grade math. If you want to know how long will it take to double any quantity that grows at a fixed rate, take the number 70 and divide it by the rate of growth.[2] This is called the doubling time:

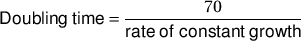
Let us go back to our example. Growth was 7% per year. It did not sound too impressive before, did it? Now, take 70, divide it by 7, it gives us 10. That means that circa every 10 years the amount of money we owe to the bank will double.

That looked easy enough, did not it? Well, that is because it is. It is a simple calculation, one that a 10-year old can do without breaking a sweat, and yet most politicians, policy-makers, urban planners, and economists worldwide fail to understand it. To be fair, any economist must have taken a statistics course at university, and the rule of 70 (or one of its variations[3]) is widely known among academics, so they know about it. But while the calculation may be easy to do, the implications of doubling over time are far less obvious and very misunderstood.

So far we have seen what it takes to double the principle. Now, let us explore the effect of this doubling over time. Suppose we borrowed $100,000 from the bank at 7% interest. As we have seen before, in just 10 years we will owe $200,000, or double the principle. But how about in 20 years? It will not be $300,000, but instead $400,000, which is two times the previous amount of $200,000 (which was itself twice the principle). How about in 30 years? You got it, $800,000! Ten more years, it is already $1.6 million. A few more years and you will owe more than you could ever make in your entire life. Luckily, most loans do not exceed the 30-year mark. But what would happen for other things, things that are not mortgage loans, and that may grow far more than 30 years? Buckle your seatbelt because we are just getting started.

3.1 EXPLOSIVE POWER

The idea of exponential growth is not new at all. In fact, it goes back thousands of years. Legend has it that when the creator of the game of chess (some say it was an ancient Indian mathematician[4]) showed his invention to the ruler of the country, the king was so pleased that he gave the inventor the right to name his prize for the invention. The man, who was very wise, asked the king this: that for the first square of the chess board, he would receive one grain of wheat, two for the second one, four on the third one, and so forth, doubling the amount each time. The king, who had no idea of the power of the exponential function, quickly accepted the inventor’s offer, even getting offended by his perceived notion that the inventor was asking for such a low prize, and ordered the treasurer to count and hand over the wheat to the inventor. Few days pass by, the inventor receives only a handful of grains, and the king is somewhat baffled. After a week, the inventor started bringing home big bags of wheat. A few days after that…you see where this is going, right? We start with 1, the next day we double, so we have 2 grains. The next day is 4 grains. Then 8, 16, 32, 64, 128, 256, 512... in just 10 days, we went from 1 to 1,024 grains. 10 doublings give you a 1,000-fold increase from the original amount. Here is where things start to take off. 10 more doublings and you have 1 million grains. 10 more: 1 billion grains. Then 1 trillion... we can stop right there. We already passed the limit of our brain. Figure 1.1 is a graphical representation to describe the process:[5]

Figure 1.1: Top left, it begins with 1 grain. It goes on to the right with 2, 4, 8, 16... then numbers grow too big, we start to use the binary notation: K=kilo (1 thousand), M = Mega (1 million), G = Giga (1 billion), T = Tera (1 trillion), P = Peta (1 quadrillion), E = Exa (1 quintillion).

On the entire chessboard there would be:

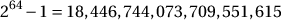
grains of wheat weighing 461,168,602,000 metric tonnes. That must be a lot of wheat. But just how much wheat are we talking about? More then the king could afford, I can tell you that. In fact, it would be a heap of wheat larger than Mount Everest, earth’s highest mountain, with a peak at 8,848 metres (29,029 ft) above sea level. This is around 1,000 times the global production of wheat in 2010 (464,000,000 metric tonnes). That is a lot of wheat. It might very well be more than the entire production of wheat in the history of humanity, combined.

As impressive and incredible as it may sound, we have to remember that this is not just an intriguing fairy tale that we like to tell. It is not merely an intellectual curiosity. It is a story that helps us understand the world around us, and make predictions about how we should go about building our future.

Over the past three years I have given a number of talks, and often I like to play a little game with the audience, to test their comprehension of an exponential increase. Most people do not get it right away, even among the most educated of audiences, so do not feel bad if it does not come to you on the spot.

Imagine an empty glass of water (technically a glass is made of glass and is full of air, but please bear with the limitations of our language). Place some bacteria inside, and let them replicate, by giving them food. The replication process is such that the number of bacteria doubles every minute. After 60 minutes, the glass is full, and since there is no more space left for food, the bacteria die. The question is: what percentage of the glass did the bacteria fill after 55 minutes?

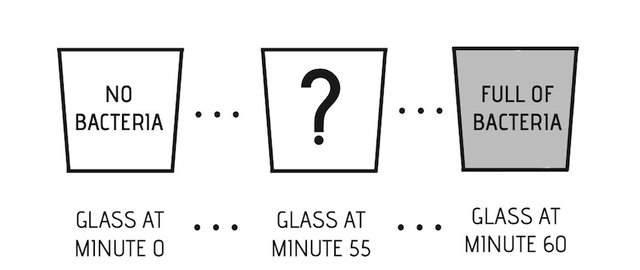
Figure 1.2: On the left, at minute zero, there are no bacteria in the glass. On the right, after a certain amount of doublings, the bacteria filled the whole thing. But what happens at minute 55 (in the centre)?

How much would you say? Take a pencil and use this empty page to scribble, sketch, and do some calculations. The answer is on the next page, but I strongly encourage you to have fun and try it out for yourself first.

Scribble, sketch, and have fun!

I hope you did try to solve it yourself, because learning is so much more fulfilling when it is interactive. If you did not, too bad for you. ☹

In truth, the bacteria have only filled 3.125% of the glass. But how can this be? Well it is simple. If they double every minute, and they fill the entire glass in 60 minutes, then they will have filled half the glass the minute before 60 (or 50% after 59 minutes), half of that the minute before 59 (or 25% after 58 minutes), and so on. Table 1.1 summary of the last 10 minutes, starting from the end.

Time Elapsed	Amount Filled
60 minutes	100 .000%
59 minutes	50 .000%
58 minutes	25 .000%
57 minutes	12. 500%
56 minutes	6. 250%
55 minutes	3. 125%
54 minutes	1. 563%
53 minutes	0. 781%
52 minutes	0. 391%
51 minutes	0. 195%

Table 1.1: Exponential growth of bacteria in a bottle over the last 10 minutes.

It all makes sense now, right? Suddenly it becomes clear, even obvious. Who could not get this? It is so simple, right? Apparently, it is not. The most common replies I get are between 50% and 90%. Even college graduates typically get it wrong. And let us not talk about politicians.

We will come back to this in the Appendix, with some real-world examples. For now, I think it is safe to say that we all understand what steady growth means. Let’s now see how this applies to our main focus in the next chapter: information technology.

Chapter 3 Notes

1. Sustainability 101: Arithmetic, Population, and Energy, Albert Bartlett. http://jclahr.com/bartlett/
2. The reason is for this quite simple. 70 is approximately 100ln(2). So, doublingtime 100ln(2) 69:3. If you want the time to triple the formula is: triplingtime 100ln(3) 109:8. The time to grow n-times is 100ln(n).
3. Rule of 70. Wikipedia. http://en.wikipedia.org/wiki/Rule_of_70
4. According to other accounts, it was a legendary Dravida VellalarDravidian peoples is a term used to refer to the diverse groups of people who natively speak languages belonging to the Dravidian language family. Populations of speakers of around 220 million are found mostly in Southern India. Vellalars (also, Velalars, Vellalas) were, originally, an elite caste of Tamil agricultural landlords in Tamil Nadu, Kerala states in India and in neighbouring Sri Lanka; they were the nobility, aristocracy of the ancient Tamil order (Chera/Chola/Pandya/Sangam era) and had close relations with the different royal dynasties named Sessa or Sissa. There exist many different variation of the same story, one set in the Roman Empire involving a brave general and his Cæsar, another with two merchants at the market, all different situations producing the same result. source
5. Image courtesy of Wikipedia.

---

Publication on Steemit

I decided the share my book, the result of years of work and research, with the Steemit community for free, to spark a debate and to raise awareness on this pressing social issue.

Previous Chapters

• Chapter 1: Unemployment Today https://steemit.com/books/@federicopistono/hi-steem-community-i-am-the-author-of-robots-will-steal-your-job-but-thats
• Chapter 2: The Luddite Fallacy https://steemit.com/book/@federicopistono/chapter-2-the-luddite-fallacy-or-robots-will-steal-your-job-but-that-s-ok

If you'd like to support my work, you can:

• upvote this post :)
• follow me @federicopistono to get updates on your personal feed
• get a copy of the book.

---

Where to find me:

• http://federicopistono.org
• https://twitter.com/federicopistono
• https://fb.com/federicopistono.page