Mastering the Differential Calculus - It's All Routine

rufu (52)in #blog • 6 years ago (edited)

Mastering the calculus depends upon understanding the process of differentiation. Here we look at this topic in its most basic dress.

Of the two branches of the calculus, differential and integral, the former lends itself quite well to routine. That is, once the basic rules of differentiation are learned, the student can master this arm quite well. Strength in algebra is required and the ability to make manipulations with and navigate through some complicated expressions. Yet overall, taking derivatives---which is what differential calculus is made of---is within reach of practically any motivated student.

source

The Derivative

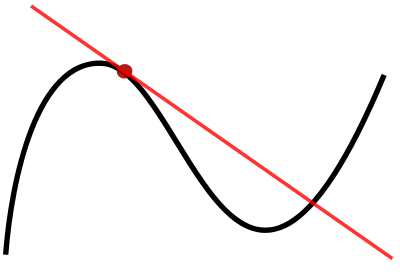
The derivative is the instantaneous rate of change of one variable with respect to another. If we think of the function y = f(x), then the derivative of y with respect to x is expressed as y' or f'(x). If you recall the concept of slope from algebra, then the derivative translates to the slope of the tangent line drawn to a curve at a particular point. To visualize what the derivative really means, imagine a plane flying along a predetermined curve drawn out in the sky. Even though the flight of the plane is occurring in three dimensions, we shall project this situation onto two: the up and down motion of the plane corresponds to the vertical, or y-axis, and the forward motion of the plane corresponds to the horizontal, or x-axis.

If we took a snapshot of the plane at a particular instant as it traversed this curve, we can then draw a tangent line to the curve at that point in time. If the curve that the plane was traveling was given by y = f(x), then the slope of this tangent line would be given by y = f'(x).

Calculus in Less Than Two Minutes

I would often show my first year algebra students that I could teach them some calculus in less than two minutes. What I was specifically referring to was the process of taking a derivative. More specifically, I would show them how to use the power rule to take a derivative of a cubic function such as y = x^3. The derivative y' is of course 3x^2, which is obtained by taking the exponent, placing it in front of the x-term, and then dropping the original power by 1. What could be easier?

My students would marvel at this display of mathematical bravado. Yet the reality is that taking derivatives is quite routine. If you understand the few simple rules, then the rest is all mechanics---nothing more. The process of differentiation should thus never be construed as something that is too difficult to learn.

Taking Derivatives

Let's look at some of the basic derivative rules and see how easy it is to apply them to some common functions.

Derivative of a Constant: If y = c, as y = 3, then y' = 0. That is the derivative of any constant is 0.

Derivative of the Power Function: If y = x^n then y' = nx^(n-1). Thus if y = x^5, then y' = 5x^4.

Derivative of a Composite Function (Chain Rule): If y = f(g(x)) then y' = f'(g(x))g'(x). For example, if y = (3x^2 + 1)^4, you can think of g(x) = 3x^2 + 1 and f(x) = x^4. Then y' = {4(3x^2 + 1)^3}(6x).

There are other rules for taking derivatives, yet the three previous ones are among the most important. The key is that if you can understand these three, the rest come naturally and without difficulty. To reiterate, taking derivatives is nothing more than routine procedure. Once this branch of the calculus is mastered, the other branch---integral calculus---is easier to tackle.

References