SLC S22W4//Linear and Quadratic equations

Greetings ,

This is @artist1111. I hope you are all doing great! Today, I am super excited to sharing my assignment on Linear and Quadratic Equations. The lecture was both engaging and informative, and truly I am thrilled to showcase my learning and the work I’ve completed.

_{Canvas source}

Explain difference between linear and quadratic equations. Provide examples of each type of system of equation and describe their general forms.

Difference Between Linear and Quadratic Equations

Linear and quadratic equations differ primarily in the degree of their variables and their graphical representation.

Linear Equations

A linear equation is equation where the highest power of the variable which is 1. These represent straight a lines on a graph. They involve a constant rate of the change and it typically describe simple, proportional relationships.

• General Form:
  ax + b = 0
  where a and b are constants, and x is the variable.

• Example of a Linear Equation:
  2x + 3 = 7
  Solving for x:
  x = 2

• System of Linear Equations:
  A system involving multiple linear equations:

y=x^2 +2x−3

These can be solved using substitution, elimination, or graphing methods.

---

Quadratic Equations

A quadratic equation is one where the high power of the variable and it is 2. These equations form a parabola when graphed & are often used to model curved relationships such as trajectories or areas.

• General Form:
  ax^2 + bx + c = 0
  where a ,( b , and c are constants, and x is the variable.

• Example of a Quadratic Equation:
  x^2 - 4x + 4 = 0
  Solving using factoring:
  (x - 2)(x - 2) = 0
  x = 2

• System of Quadratic Equations:
  A system with at least one quadratic equation:
  y = x^2 + 2x - 3
  y = 2x + 1

These systems can be solved using substitution or graphing to find points of intersection.

---

Key Differences

Aspect	Linear Equations	Quadratic Equations
Degree	1	2
Graph	Straight Line	Parabola
Rate of Change	Constant	Varies
Form	( ax + b = 0 )	( ax^2 + bx + c = 0 )
Example	( 3x + 2 = 8 )	( x^2 - 5x + 6 = 0 )

Describe methods for solving quadratic equations and share pros and cons for each method.

Quadratic equations can be solved using several methods, each with its own advantages and disadvantages. Here’s an overview of the main techniques:

---

1. Factoring

This method involves expressing the quadratic equation as a product of two binomials.

---

2. Quadratic Formula

The quadratic formula is a universal method applicable to all quadratic equations:

--

3. Completing the Square

This method involves rewriting the equation into a perfect square trinomial.

---

4. Graphing

Graph the quadratic equation and identify the points where it crosses the x-axis (if any).

• Process:
  Graph (y = ax^2 + bx + c).
  Find the x-intercepts, which are the solutions.

• Example:
  Graph (x^2 - x - 6 = 0):
  The graph crosses the x-axis at (x = -2) and (x = 3).

---

Pros and Cons

Method	Pros	Cons
Factoring	Simple and quick for factorable equations.	Limited to equations that are easily factorable.
Quadratic Formula	Universal and works for all quadratics.	Lengthy for simple equations; requires computation.
Completing the Square	Useful for equations that are not factorable.	Time-consuming and requires additional steps.
Graphing	Visual and intuitive for understanding solutions.	Not precise for non-integer solutions.

Solve for linear equation 3x + 2 = 11 and show value of x?

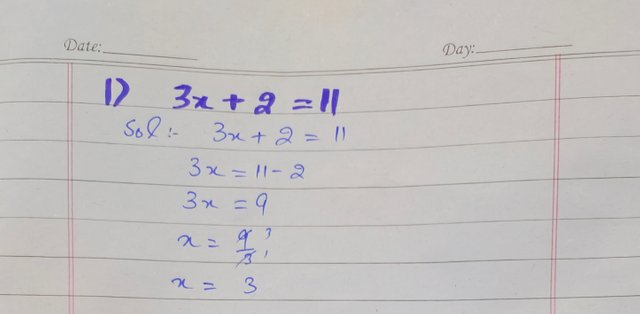Solve for this quadratic equation x^2 + 2x - 6 = 0.

Scenario 1: Solving for the Remaining Money

Problem:

Ali has $15 for snacks and spends $3 on a pack of chips. The equation is:
x + 3 = 15

where x is the money Ali has left.

Step-by-Step Solution:

1. Start with the given equation:

   x + 3 = 15

1. Subtract 3 from both sides to isolate ( x ):

   x = 15 - 3

1. Simplify:

   x = 12

Ali has $12 left after buying the pack of chips.

---

Scenario 2: Maximum Height Reached by the Ball

The ball reaches a maximum height of 20 meters after 2 seconds.