Steemit Learning Challenge Season 22 Week 2//Equations and inequalities

Explain difference between linear and quadratic equations. Provide examples of each type of equation and describe their general forms.

Understanding the difference between linear and quadratic equations is like spotting the difference between a straight road and a rollercoaster. One is predictable and straightforward, while the other loves curves and thrills! Let’s dive in:

Linear Equations

Linear equations are the calm, no-drama type. They represent straight lines when graphed and follow a simple, predictable path. The highest power of the variable is always 1. Think of them as the “one-step-at-a-time” kind of equations.

General Form:
ax + b = c
Here, ( a ), ( b ), and ( c ) are constants, and ( x ) is the variable.

Example:
2x + 3 = 7
This equation tells us, “Hey, just solve for ( x ), and you’re good!” (Spoiler: ( x = 2 )).

Fun Thought: If a linear equation were a person, it would wear a suit and follow the rules. No twists, no surprises.

---

Quadratic Equations

Quadratic equations, on the other hand, are the adventurous type. Their graphs form a curve called a parabola, which could be a happy smile (opens upward) or a deep frown (opens downward). The highest power of the variable here is 2, which makes them a bit more dramatic.

General Form:
ax^2 + bx + c = 0
Here, ( a ), ( b ), and ( c ) are constants, and ( x ) is the variable.

Example:
x^2 + 4x + 4 = 0
This equation says, “Hold on tight! You might need to factorize or use the quadratic formula to solve me!” (Hint: The solution is ( x = -2 )).

Fun Thought: If a quadratic equation were a person, it would love rollercoasters and always carry a quadratic formula cheat sheet.

---

Key Difference

• Graph Shape:

• Linear: Straight line

• Quadratic: Parabola (U-shaped)

• Degree of Variable:

  • Linear: Highest power is 1
  • Quadratic: Highest power is 2

• Example Scenario:

  • Linear: “I earn $10 per hour.” (Simple and linear!)
  • Quadratic: “The height of a ball I throw depends on time squared.” (Physics drama at its best!)

Final Thought: Whether you like the simplicity of linear equations or the flair of quadratic ones, both are vital tools in algebra. It’s like choosing between a steady road trip and a thrilling amusement park ride—each has its charm!

Describe two different types of inequalities(Which are not explained in course). Provide examples of each type of inequality and explain how to solve them.

Inequalities are like puzzles that express the relationship between quantities that aren't equal. While linear and compound inequalities are common, there are other fascinating types of inequalities worth exploring. Let’s dive into absolute value inequalities and rational inequalities—two intriguing variants that bring their own unique challenges and methods of solving.

---

1. Absolute Value Inequalities

Absolute value inequalities involve expressions wrapped inside the absolute value symbol (|•|), which represents the distance of a number from zero on the number line. These inequalities express conditions like "how far" a value can stray from a certain point.

Example:

Fun Fact: Absolute value inequalities are like setting boundaries—they keep variables within or beyond a specific range.

---

2. Rational Inequalities

Rational inequalities involve expressions with fractions where the numerator and/or denominator contains variables. These inequalities determine where a fraction is greater or less than a specific value.

General Form:

How to Solve:

1. Find Critical Points: Set the numerator and denominator equal to zero to find the critical points.
2. Test Intervals: Divide the number line into intervals based on the critical points. Test each interval to determine where the inequality holds true.
3. Exclude Undefined Values: Ensure the denominator is never zero.

Example:

Fun Fact: Rational inequalities often feel like balancing acts—they test your ability to manage multiple intervals and restrictions.

---

Conclusion:
Both absolute value and rational inequalities add depth and variety to the world of algebra. While absolute value inequalities focus on distance and boundaries, rational inequalities challenge you to think critically about fractions and intervals. Mastering them not only sharpens your problem-solving skills but also makes you appreciate the creative side of mathematics!

Solve the following linear equation: 2x + 5 = 11.Show step-by-step solution and share its practical example of how this equation can be applied in real life scenario.

(You are required to solve this problem at paper and these share clear photographs for adding a touch of your creativity and personal effort which should be marked with your username)

In general way ;

Practical Example:

Scenario:
Imagine you are planning a party and want to rent chairs for your guests. Each chair rental costs $2, and you have already paid a $5 booking fee. If your total bill is $11, how many chairs did you rent?

Solution Using the Equation:
Let (x) represent the number of chairs rented. The total cost is given by:

2x + 5 = 11

From the solution above, ( x = 3 ).

Answer: You rented 3 chairs.

Real-Life Insight:
Linear equations like this are common in budgeting, shopping, and resource allocation. They help us determine unknown quantities when costs or other factors are involved!

Task 4: Scenario: Number of gallons Ashley needs.
Suppose there's a person named Ashley who is planning a road trip from City A to City B. If I talk about distance between two cities then it's around 240 miles. Ashley's car fuel tank can hold 12 gallons Suppose that his car gets 20 miles for each gallon then how many gallons of gas may Ashley purchase if he already has 2 gallons in his tank and he wants to have a minimum of 1 gallon left over when he arrives at City B?

In Tom's bakery, mathematics comes to life through equations and inequalities. Let's analyze how Tom can optimize his resources for baking chocolate cakes while managing ingredient constraints.

Equation: Calculating Maximum Cakes

Equations represent exact relationships. Here, Tom’s equation helps determine the exact number of cakes he can bake. For each cake, the recipe requires:

• 2 cups of flour
• 1 cup of sugar
• 0.5 cups of cocoa powder

Tom has 10 cups of flour, 8 cups of sugar, and 4 cups of cocoa powder. By setting up constraints:

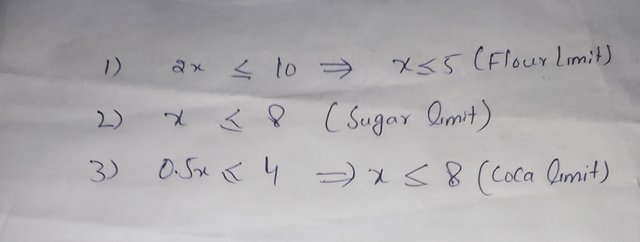Flour becomes the limiting factor, and Tom can bake a maximum of 5 cakes. This showcases the principle of constraints in linear equations, where the smallest value dictates the solution.

---

Inequality: Ensuring Minimum Goals

Inequalities provide flexibility, defining ranges instead of exact values. Here, Tom wants to bake at least 6 cakes with 8 cups of sugar. Each cake needs 1 cup of sugar, so:

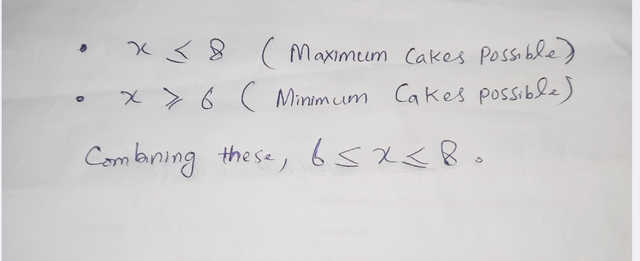Tom can bake 6 to 8 cakes, meeting his goal without exceeding his sugar limit.

Additionally
Suppose Tom adjusts the recipe to use 1.5 cups of flour per cake instead of 2 cups. The new flour constraint becomes:

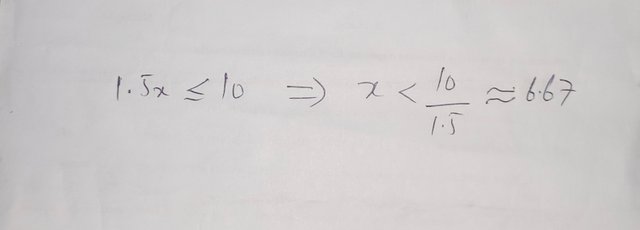Now, Tom can bake 6 cakes, slightly increasing output without exceeding any limit.

---

Mathematical Insights

This scenario demonstrates the interplay between equations (fixed solutions) and inequalities (flexible ranges) in resource management. Tom’s flour constraint reflects the binding constraint concept in optimization, while sugar aligns with feasibility analysis. Such calculations are invaluable in production planning, ensuring efficiency and customer satisfaction in real-life business operations.